Too many people think Math is Dull. 

Astounding Math Stories exists to show you

the kinds of weird things that happen when you do math.

These Astounding Stories also illustrate some important ideas in math,

so this blog also has a Secret Educational Agenda.

 

Table of contents

The unreliability of numerical evidence

A conversation recorded by the Six Winged Seraph, a certified member of the Society of Recording Angels

18 December 2008

Gyr:  I’ve just discovered something . The Perrin function on the natural numbers is defined inductively this way:

                                          

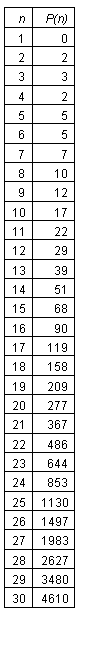
The table shows the first 30 values.  You can check that for these values, if n divides P(n), then n is prime and if n doesn’t divide P(n), then n is not prime.  For example 11 divides P(11) and 11 is prime, but 12 does not divide  P(12) and 12 is not prime.   In fact, this checks out through the first couple of hundred thousand integers:  always if n divides P(n), then n is prime, and conversely.

 

Gimblos:  Sweet.  Then isn’t that connection between n dividing P(n) and n being prime always true?  Because the definition of the Perrin function involves only small integers, so if something is going to go wrong it’s sure to go wrong before 200,000.  I mean, suppose I were a biologist and I discovered a new species of frog.  Suppose I dissected several specimens and discovered that each one had two livers.  Then I could write a paper saying that I had discovered a new species of frog that had two livers. A paper like that could get published in The Journal of Frogs (or something) and my reputation would be enhanced.  Maybe I would even get a tenure-track job.  But the referees of the journal wouldn’t expect me to dissect over 200,000 specimens before they accepted the paper!  Or even 100.

 

Gyr:  Ah, but biology isn’t mathematics!  Mathematics is a Realm of Pure Thought Unsullied by Experimental Evidence.  (See Note 1.)  In math if you want to know that some statement is true you have to prove it.

 

Gimblos:  Well, has anyone proved it?

 

Gyr:   Well, no, because it is false. (Note 2.)  In fact

 271,441 divides P(271,441),

but 271,441 is not prime (it is 521²).      

 P(271,441) has 33,150 digits.  It is an example of a composite number for which n divides P(n).  Mathematics is full of .  You need to be paranoid when you do math research. 

MORAL:  In math, checking a mere few hundred thousand cases

never tells you everything.

Notes

added by the Six Winged Seraph

Note 1

This is know as ROPTUBEE.  It is always printed in purple prose so you will know how important it is and how exalted mathematicians are. 

Note 2

It has been proved that for any natural number n, if n is prime, then n divides P(n), so the converse is in fact true for all n.

If you want to learn more:

There is research linking Perrin pseudoprimes and Fermat’s Little Theorem.  Both the sources listed below get into that, via links in the case of the MathPages site.

MathPages article on Perrin’s sequence

Frobenius Pseudoprimes, by John Grantham