abstractmath.org 2.0
help with abstract math
Produced by Charles Wells Revised 2017-04-13
Introduction to this website website TOC website index blog
UNDERSTANDING MATH
In mathematics you don’t understand things, you just get used to them. –John von Neumann
This
page is intended to raise your consciousness about the many ways there are to
understand “understand” in math. A link to each chapter about
understanding math is given here with some comments about the ideas expressed
in it.
- One
piece of information you must have about a math concept is its definition.
- Every
proof of a fact about the concept must be based on a logical chain of
reasoning starting with the definition.
- The
definition is one path to understanding the concept but not the only
one.
- Mathematical
objects are what mathematics is “about”.
- The
number $42$ is a math object.
- The set of even positive integers is a
math object. Even though it is an infinite set, it is a single math object.
- The function $f(x):={{\sin }}x$ is a
math object. Its value can be computed at many different numbers but it is a single,
static math object. You can visualize going along it from left to right, which makes you go up and down over and over between $1$ and $-1$, and it is useful to visualize it that way (see Images and Metaphors), but it is a single,
static math object.
- We talk
and think about math objects in some of the same ways that we talk about
physical objects, but in other ways they are not like physical objects.
A mathematical structure is a special kind of math
object defined as a set with some associated objects called structure.
Equivalence relations, partitions, groups and topological spaces are examples of
mathematical structures.
“Representation”
and “model” have several related meanings.
- We can represent
the number $42$ in binary notation as $101010$ and in hex as $2A$.
- We can represent
a function by its formula or by its graph, or sometimes by a listing of its
values.
- When we
think about some math objects we may have a mental representation
of them (like whooshing up and down on the graph of the sine function).
- We can model
the trajectory of a ball by a function.
- A group
is a model
- We think of a function that has a positive derivative as “going up”. But the
function isn’t really going up, it just sits there like your
pet rock.
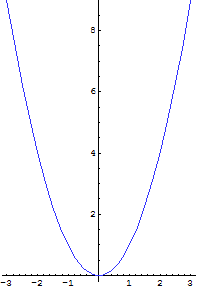
- We think of the parabola $y={{x}^{2}}$ as like a bowl whose sides go up
forever. But “forever” is a metaphor. There is no time involved.
- Besides,
we may forget that the sides of the parabola go out in the positive and negative x
direction “forever”, too (every vertical line cuts
the graph!), even though the picture looks like they are too steep to do that.
As these examples illustrate, we think
about math objects in terms of images and metaphors that we
have developed out of our experience with them. These are valuable insights
but they generally cannot be used to prove theorems about them.
There is a way of thinking about any math object that helps when you are trying to prove something: the rigorous view of math objects,
also discussed in this chapter.
When mathematicians consider a math object they are typically interested
in two different aspects of the object:
- What is
it? What properties does it have? How is it different from
other math objects? I want a conceptual understanding of the object.
- How do
I compute with it? How do I find a value of the object (if that makes
sense)? How to I tell how big it is (in some sense of big)? How do I determine in an efficient way what properties it
has?
Proofs
can have a conceptual side and a computational side too.
- A conceptual proof helps you understand why the statement is true.
- A
computational proof may be easier to check systematically to see if it is
correct, and to automate using some suitable computer program.
This catch-all chapter talks about
several special phenomena that are involved in understanding math.

This work is licensed under a Creative Commons Attribution-ShareAlike 2.5 License.

